Graphene is probably the greatest inspiration in current solid-state research. Many unique properties of graphene are due to its peculiar electronic structure, characterized by linear band dispersion and crossings of these bands at the Fermi level (the Dirac points). The linear dispersion near the Fermi level leads the charge motion being described by the Dirac Hamiltonian for massless particles, rather than by the standard Schrödinger Hamiltonian used for conventional parabolic bands [Wehling2014].
The search for other materials, where similar physics would be observed, has led to the theoretical predictions and eventually to the experimental discovery of topological insulators, see Ref. [Moore2010] for a review on early TIs history. Unlike its bulk, the surface states of topological insulators are conducting and the electronic structure of these states is similar to the electronic structure of graphene. The search for other types of materials with Dirac physics also lead to theoretical prediction of three-dimensional analogues of graphene [Murakami2007, Wang2012, Wang2013].
These materials, usually referred to as three-dimensional Dirac semimetals, possess the linearly dispersed bands in their bulk (and in all three dimensions of the momentum space), while the Dirac physics in TIs is locked to the surface, i.e. to low dimensions. Handling with bulk electrons can be rather advantageous for potential utilization of the remarkable properties of Dirac fermions, such as extremely high mobility and vanishing effective mass. Currently, bulk topological Dirac points are well confirmed in such materials as, e.g., Na3Bi, Cd3As2, SrMnBi2, etc. [Armitage2017].
A peculiar case of linear band crossing attracts special interest. This is the case of Weyl semimetals, which can be realized if either inversion or time-reversal symmetry is broken. A Weyl semimetal is a 3D system which band structure contains pairs [Nielsen1981] of linear-band crossings (called Weyl points). Each Weyl point can be assigned a chirality (handedness) χ that takes values +1 or -1. Half the Weyl points have positive, the other half negative chirality. The only way to annihilate a Weyl point is if two points of opposite chirality meet together. The Weyl state has recently been confirmed in TaAs, NbP, WTe and many other compounds [Armitage2017]. The splitting of the Weyl points in the reciprocal space is the source of the so-called chiral anomaly, i.e. a violation of the charge conservation law for electrons of a given chirality near a Weyl point. This anomaly is a source of the most unusual properties of Weyl semimetals.
Potential application opportunities of Dirac and Weyl semimetals are calling for basic investigations on the unveiled Dirac physics in these systems. It is important to recognize that the properties of Dirac electrons become significant only in the low-energy limit [Wehling2014]. Thus, one has to study the low-frequency excitations in order to learn about the peculiar dynamics of Dirac electrons. At PI1 we explore the low-energy (in practice, terahertz and infrared) electrodynamics of the three-dimensional Dirac materials. Optical studies at terahertz and far-infrared frequencies provide direct access to excitations at very low energy scales (a few meVs), which are hard to access by other methods, such as, e.g., ARPES.
Below we give a few examples of our recent measurements.
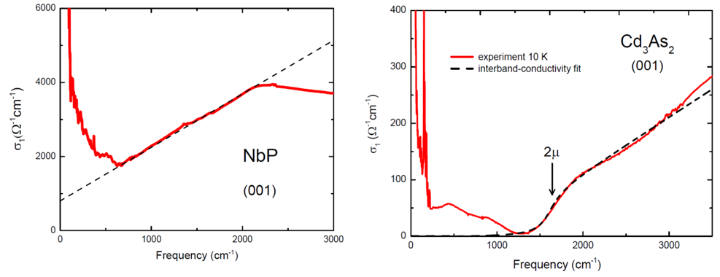
FIG. 1. Examples of optical conductivity spectra of Weyl and Dirac semimetals.
Left panel: optical conductivity of NbP at T = 300 K. The linear in frequency conductivity is due to transitions between 3D bands with linear dispersion. At low frequencies, the spectrum is strongly affected by intraband (Drude) conductivity. This leads to the positive intercept of the interband-conductivity extrapolated to ω = 0 (dashed line) with the vertical axes and masks the position of the chemical potential.
Right panel: optical conductivity of Cd3As2 at T = 10 K [Neubauer2016]. The position of twice the chemical potential is marked with an arrow (the step at 2μ is smeared out because of the scattering). Phonons and intraband Drude conductivity, dominating the low-frequency part of the spectrum, are not included in the model fit. Deviations from a perfect linear σ(ω) at ω > 2000 cm-1 are due to the band-structure peculiarities of Cd3As2, see e.g. [Jeon2014, Neubauer2016].
Optical measurements for both compounds are made on the (001) crystallographic plane; no in-plane anisotropy is detected.
In all compounds under examination, we found some portions of the interband optical conductivity, σ(ω), to rise linearly with increasing frequency, as plotted in Fig. 1. The linearity of the interband σ(ω) reflects the linearity of the 3D bands [Hosur2012] and is widely considered as an indication of the 3D Dirac/Weyl semimetal state. For the simplest case (chemical potential is at the Weyl/Dirac point, i.e. μ = 0), one has [Hosur2012]:
where NW is the number of (non-degenerate) Weyl points, e is the elementary charge, and vF is the Fermi velocity. Hence, from the obtained conductivity spectra, the Fermi velocity can be directly extracted (more elaborative analysis for anisotropic/titled bands with arbitrary position of μ is given e.g. in Ref. [Carbotte2016]). The onset of the linear conductivity is related to the position of the Fermi level [Ashby2014].
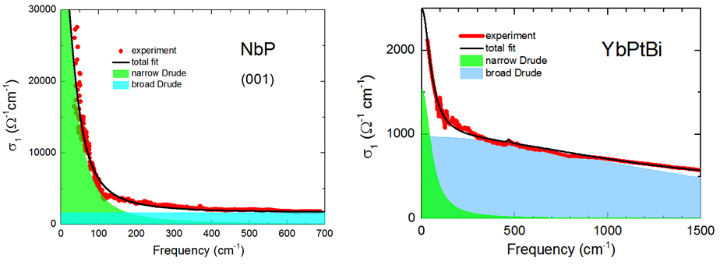
FIG. 2. Typical low-frequency (far-infrared) conductivity of Weyl/Dirac semimetals studied.
Left panel: NbP (same data as in Fig. 1, but on a different scale).
Right panel: Weyl-semimetal candidate YbPtBi at 300 K (it has cubic symmetry and isotropic optical conductivity). Half-widths of the Drude modes correspond to the scattering rates.
Note that for lower temperatures, the narrow Drude contributions become significantly narrower.
We have found that the low-frequency (THz/far-infrared response) of all studied so far by us Dirac and Weyl semimetals reveals a very narrow Drude-like band with a very low scattering rate, typically around 1 to 10 cm-1. This narrow band is the optical response of the highly mobile carriers inherent to the Dirac/Weyl semimetals. For some compounds, e.g. for NbP, TaAs, and YbPtBi, the narrow Drude band is accompanied by another Drude band with a much larger scattering rate, typically around a few hundreds cm-1. This second Drude term is due to less mobile carriers, i.e. other bands with presumably parabolic dispersion also contribute to the low-frequency response (as well as to dc transport). In Fig. 2 we show examples of the low-frequency optical conductivity, dominated by two Drude (intraband) contributions.
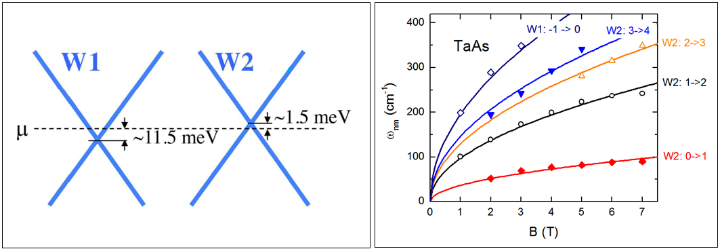
FIG. 3. Left panel: Sketches of the bands near the Weyl points in TaAs, after [Zhang2016]. TaAs is known to have Weyl nodes of two types (commonly dubbed as W1 and W2). The nodes have different Fermi velocities. The energy separation between W1 and W2 is around 12 to 13 meV, while the position of the chemical potential may somewhat vary from sample to sample.
Right panel: Frequencies of the resonance transitions between different LLs (n→m) versus magnetic field applied, as obtained from our magneto-optical reflectivity measurements of TaAs. Dots represent experimental data; lines are fits with Eq. (2) and two different Fermi velocities for W1 and W2. The values of vF obtained from the fits are in excellent agreement with calculated values from Ref. [Arnold2016].
In Weyl and Dirac semimetals, the frequency ωnm, where the resonance absorption between two Landau levels (LLs) with indices n and m takes place, is related to the applied magnetic field is the following way:
ωnm = En - Em = sgn(n)vF √2|n| heB/(2πc) - sgn(m) vF √2|m| heB/(2πc) (2)
i.e. ωnm is proportional to √B. This proportionality is a hallmark of linearly dispersing bands; it is remarkably different from the common linear behavior of the resonance frequency in parabolic bands, where the LLs are spaced equidistantly.
In the right-hand panel of Fig. 3, we show our results on optically detected transitions between different LLs for the Weyl semimetal TaAs. The resonance frequencies do show the square-root behavior, as expected from Eq. (2).
Armitage2017 - N.P. Armitage et al., arXiv:1705.01111
Arnold2016 - F. Arnold et al., Phys. Rev. Lett. 117, 146401 (2016)
Ashby2014 - P. E. C. Ashby and J. P. Carbotte, Phys. Rev. B 89, 245121 (2014)
Carbotte2016 - J. P. Carbotte, Phys. Rev. B 94, 165111 (2016)
Hosur2012 - P. Hosur, et al., Phys. Rev. Lett. 108, 046602 (2012)
Jeon2014 - S. Jeon et al., Nat. Mater. 13, 851 (2014)
Moore2010 - J. E. Moore, Nature 464, 194 (2010)
Murakami2007 - S. Murakami, New J. Phys. 9, 356 (2007)
Neubauer2016 - D. Neubauer et al., Phys. Rev. B 93, 121202 (2016)
Nielsen1981 - H. B. Nielsen and M. Ninomiya, Phys. Lett. B 105, 219 (1981)
Wang2012 - Z. Wang et al., Phys. Rev. B 85, 195320 (2012)
Wang2013 - Z. Wang et al., Phys. Rev. B 88, 125427 (2013)
Wehling2014 - T. O. Wehling et al., Adv. in Phys. 63, 1 (2014)
Zhang2016 - C. Zhang et al., Nat. Commun. 7, 10735 (2016)

Artem Pronin
PD Dr.Optical studies of topological materials